BERNOULLI CENTER
Lausanne (Switzerland)
JULY 17-21, 2023

Scientific Overview

The Bernoulli Center at EPFL (Switzerland) will host the third workshop for Women in Computational Topology.

These workshops are designed to strengthen the computational topology community by bringing together women as well as gender-diverse researchers at various stages in their careers (from graduate students to senior researchers) and from across the world, fostering research, collaboration, and mentorship between members of gender minorities in computational topology, offering them networking and research opportunities in mathematics and computer science.
Participants will spend one week working together in small groups to solve one of a selection of open questions in computational topology: WinCompTop participants will begin generating new results in collaboration with other participants.

To achieve this goal, participants will start working together remotely before the workshop itself to prepare the background and, following the workshop, the research network will be maintained and strengthened by publishing a proceedings volume and organizing follow-up conferences or reunions for participants and other researchers in the area. Mentoring and professional development will happen both formally and informally.

Preference for covering local expenses will be given to graduate students and early career researchers.

Organizers and contacts:

• Erin Wolf Chambers (St. Louis University), erin.chambers@gmail.com
• Heather Harrington (University of Oxford), harrington@maths.ox.ac.uk
• Kathryn Hess (EPFL), kathryn.hess@epfl.ch
• Claudia Landi (University of Modena and Reggio Emilia) clandi@unimore.it

Acknowledgements:

This workshop is supported in part by the NSF award number 2317401, titled: “NSF Travel: Third Workshop for Women in Computational Topology”.

View list of participants

Programme

• The workshop is scheduled to begin on Monday, July 17 2023.
• Participants are encouraged to arrive on Sunday, July 16, 2023.
• The workshop will conclude in the late afternoon of Friday, July 21, 2023

Here is the detailed agenda:

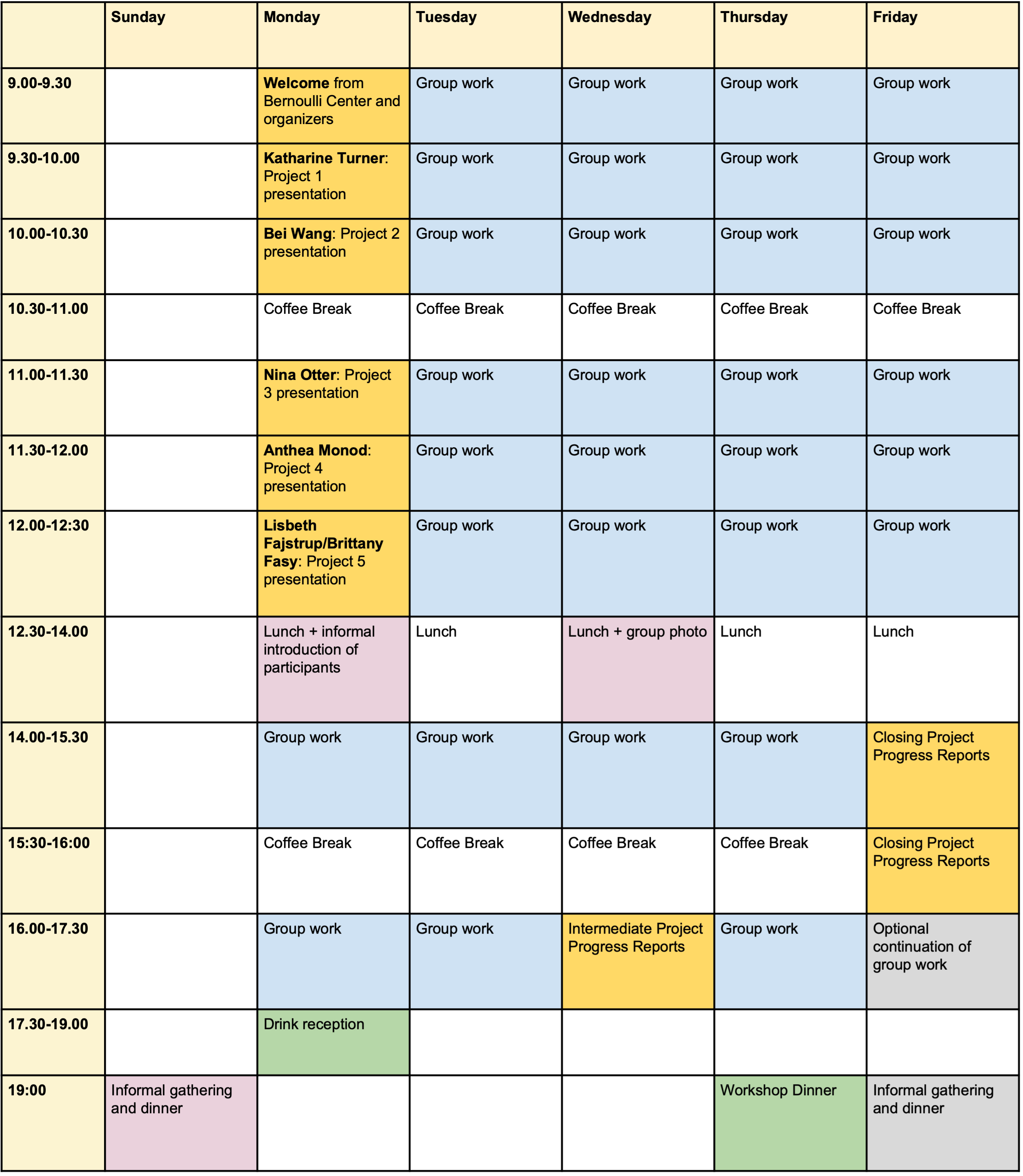
Selected topics and group leaders:

The specific research groups, along with the group leader submitting the idea, are:

• Project 1: Decompositions of the persistent homology transform, led by Katharine Turner (Australian National University)
• Project 2: Multilevel sparsification of higher-order data, led by Bei Wang (University of Utah)
• Project 3: Studying self-similarity of complex networks with persistent magnitude, led by Nina Otter (Queen Mary University London)
• Project 4: Probabilistic Behavior of Prevalence-Augmented Barcodes, led by Anthea Monod (Imperial College London)
• Project 5: Connections between dynamic programming and directed topology, co-led by Lisbeth Fajstrup (Aalborg University) and Brittany T. Fasy (Montana State University)

Additional information on the projects:

• Project 1, on decompositions of the persistent homology transform, led by Katharine Turner (Australian National University): The persistent homology transform provides a topological way to study the geometry of rigid objects with past applications including the study of the shape of bones, cancer tumors and proteins. The decomposition of persistent homology into interval modules is well understood and we can generalise this notion of an indecomposable to a continuous map from a cover of the sphere to the space of interval modules (including the empty module as an option). The group would investigate this decomposition – proving properties, providing examples and counterexamples. There are then three distinct paths of research. One is to use these decompositions to perform semi-parametric modelling of shapes – where the parameters are the ways to construct the indecomposables. The second is the use the decompositions to define a modified version of Wasserstein distance and to perform statistical shape analysis with this new distance. The final path for research is to investigate whether we use the approximation of indecomposables to reconstruct shapes from samples of the persistent homology transform by finitely many directions.
• Project 2, on multilevel sparsification of higher-order data, led by Bei Wang (University of Utah): Simplicial complexes and hypergraphs can be used to model higher-order interactions between three or more objects in complex systems. We are interested in performing multi-level sparsification of simplicial complexes; for example, we would like to sparsify triangles and edges simultaneously while preserving their spectral properties. Such sparsification is challenging if we would like to simultaneously maintain structures across dimensions. We will also relax our structural constraints to work with hypergraphs. In addition, we are also interested in deriving formal connections between homological sparsification and spectral sparsifications.
• Project 3, studying self-similarity of complex networks with persistent magnitude, led by Nina Otter (Queen Mary University London): There have been several efforts in using techniques from topology to study self-similar structure in point-cloud data. In this project we will study self-similarity of complex networks using persistent magnitude, a new invariant in TDA that has recently been introduced by Govc and Heptworth. Persistent magnitude arises from the connection between persistent homology and magnitude homology, and it is a numerical invariant of a filtered simplicial complex associated to a data set. Recent work has explored stability properties of the persistent magnitude associated to the filtered alpha complex of a point cloud, and it can be used to study self-similar properties of point-cloud data sets sampled from fractals. In this project we propose to instead study the persistent magnitude of filtered spaces associated to complex networks, to investigate its stability properties and relationship to self-similarity measures of networks, as well as to use it to classify several real-world data sets of fungal and bacterial networks. The project would be suitable to be divided into several smaller independent projects, each one taking a more theoretical or computational approach.
• Project 4, on Prevalence-Augmented Barcodes, led by Anthea Monod (Imperial College London): In persistent homology, long bars in barcodes have been typically understood as topological “signal” while short bars are considered noise. However, recent work by Reani & Bobrowski (2021) has shown that clear topological signal in point processes can nevertheless constitute barcodes where all bars are short, giving rise to an alternative understanding of topological signal referred to as “prevalence”. This notion was presented in the context of cycle matching in image-persistent homology with repeated sampling (bootstrapping). García-Redondo, Monod and Song (2022) have further developed this concept to computational efficient settings using cohomology. In particular, they propose a prevalence-augmented barcode where the length of a bar corresponds to usual persistence, but thickness and color intensity of the bars correspond to prevalence. This project aims to study probabilistic questions about prevalence-augmented barcodes, corresponding to various metrics, filtrations, sample sizes, and samples.
• Project 5, on connections between dynamic programming and directed topology, co-led by Lisbeth Fajstrup (Aalborg University) and Brittany T. Fasy (Montana State University): A dynamic programming problem is an algorithmic approach to solving recurrence relations in a smart way: by studying the relationships between the recursive sub-problems, then iteratively solving the main problem. This has a lot of structure that has a direction: For instance, the subproblems whose solutions are needed for the next level solutions are organized in a dependency graph. The first application of directed topology and indeed the reason for developing the theory was in concurrent computing where dependency is through shared objects, and equivalence classes of executions depend on the capacity of these objects, i.e., how many parallel threads are allowed access to the object at the same time. This is modeled by cubical complexes where, e.g., a solid (or filled-in) three-cube indicates that three threads can proceed at the same time.In this working group, we investigate if there is a similar way of interpreting the dependencies in dynamic programming. When problem A needs the input of the solutions of B and C, can we give that a different geometry than when it needs B or C (but not necessarily both). In other words, give the dependency graph a higher-dimensional structure. Another question could be whether dynamic programming can be used to answer algorithmic problems in directed topology.

Participants:

• Abigail Hickok, Columbia University
• Adriana Haydeé, Contreras Peruyero Centro de Ciencias Matemáticas UNAM
• Anda Skeja, EPFL
• Anthea Monod, Imperial College London
• Barbara Giunti Graz, University of Technology
• Bei Wang, University of Utah
• Brittany T. Fasy, Montana State University
• Elizabeth Thompson, Washington State University Vancouver
• Francesca Tombari, KTH- Royal Institute of Technology
• Katharina Limbeck, TUM Munich & Helmholtz Munich
• Katharine Turner, Australian National University
• Lida Kanari, EPFL
• Ling Zhou, The Ohio State University
• Lisbeth Fajstrup, Aalborg University
• Lydia Mezrag, University of Montreal
• Maria Antonietta PASCALI, Institute of Science Information and Technologies – National Research Council of Italy
• Ines Garcia-Redondo, Imperial College London
• Nina Otter, Queen Mary University London
• Polina Turishcheva, University of Göttingen (Georg-August-Universität Göttingen)
• Qiquan Wang, Imperial College London
• Rayna Andreeva, University of Edinburgh
• Renata Turkes, University of Antwerp
• Robin Belton, Smith College
• Sanjukta Krishnagopal, University of California, Los Angeles
• Sarah McGuire, Michigan State University
• Sarah Percival, Michigan State University
• Shreya Arya, Duke University
• Tatum Rask, Colorado State University
• Wenwen Li, University of Oklahoma
• Ximena Laura Fernandez, Durham University
• Ziva Urbancic, Durham University